Inverted spectra of monochromatic rays
A supplement to the video “Monochromatic Rays of Shadow”.
(Version per 2016-09-27 )
Background
|
On April 25-26, 2016 a small group gathered in Järna with the intention to check out the splitting
of monochromatic spectral light, using the same setup as the one used in the video. Participants
were myself (Pehr Sällström) and from Oslo: Arne Nicolaisen, Morten Eide and Jan Henrik Wold. We
wanted to show that spectral green splits into yellow and cyan, under the conditions when a purple
ray does not split into blue and red. And we were successful, after some painstaking work. |
 |
Prologue
Three points of departure for the study of prismatic spectra
If you get hold of a prism, the first impulse would be to look through it on the surroundings. You will be able to observe the colour phenomena Goethe so beautifully describes in his “Beiträge zur Optik” from 1790.

This is the paradigm case for our study. Goethe used white and black to represent light and darkness. He composed and studied simple pictures based on colour materials. The observations are best made in ordinary, diffuse neutral illumination, i.e. “under daylight conditions”. The stronger illumination, the better, because then contrast increases (in absolut terms). Complementary pictures are defined by interchanging black and white areas in the pictures. This was a wise approach. What is light? What is darkness? How do you manipulate them?

Goethes experiments set the agenda: we have in principle four types of spectra, pairwise complementary,
and there are infinitely many intermediate cases between the ideal extremes, due to geometrical
conditions, such as relative distance from prism to picture etc. It was Goethe’s genius that he saw
the fundamental importance of these simple observations, especially for colour theory.
*
If the sun shines into the room you can hold the prism in the light and get bright reflexes and colour spectra projected here and there on the walls.

Such a spectre is an exotic guest. It has nothing to do with what is already painted or mounted
on the wall, and of course it is best seen on a plain white wall. Due to its brilliance it does
not belong to the same gamut of colour as the materially based colours in the room. It is perceived
as luminuous rather than illuminated. In accordance with the principle of superposition of light, it
is simply added to the already given layout of luminances.
At sufficient distance from the prism the spectre is essentially an image of the sun. It has usually
been studied in a dark chamber, where the sun shines through a small opening, close to which the prism
is placed. So did Isaak Newton, in his time.
*
An alternative to this is to place the prism on a sheet of white cardboard and in the sun, preferably in the afternoon when it stands relatively low. The prism throws a dark shadow, but also a bright band of white light with tiny coloured borders with increasing angle further away from the prism. You can place various shadow-casting objects in the way, and get typical effects.
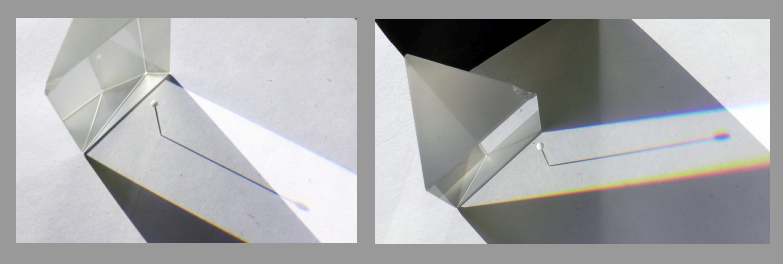
Note that you have to shield the band of ”dispersed light” from diverse illumination from the
sun and various other directions. The prismatically transformed image is not embedded in the
optical situation. It is an add-on to it. Again an example of the superposition principle.
I. Resumé of the method of producing a purple-ray
Explorative experiments in dispersed light
We have followed up the possibility of experimenting in the field of dispersed light close to a
prism surface.
To our disposal, beside a sufficiently big prism, we have plane mirrors, with the help of which
one can change the direction of illumination, so as to make possible a superposition of images, giving rise to new images. A special device is a mirror with a thin slit, an opening where it is transparent instead of reflecting (a “slit-mirror”). Further we have various opaque black plates, with or without apertures. These are used for delimiting the regions of directed light; darkness is created behind these screens. We also make use of plates of white diffusely reflecting material. This is for making the illumination visible, by observing the resultant luminance pattern on the board. Or photographing it, as the case may be.
In the continuing discourse around what we see, use is made of words and metaphors from ordinary
language and practical life. As when we speak of light and darkness, illumination, shadows, rays,
colours. Light and dark in general indicate conditions favourable, respectively not favourable,
for clear vision. But bright and dark is also used to describe the pattern of contrasting
luminances and perceived illumination conditions in a spatial situation. It remains to be
settled how these perceptual impressions are related to physical descriptions of an optical
situation. Terms such as illuminance, luminance and reflectance are more technical, pointing
at a tentative mathematical modelling of the matters at hand.
The discourse is supplemented by documentary photographs. These are not “true” (especially not
physically true) but are visually judged to be as similar as possible to what was seen with
naked eyes, in the situation they refer to.
Instead of the sun an artifical light source may be used. For instance a low voltage halogene
lamp together with a collimator, to simulate a distant point-source. This arrangement was used
in my video-film “Monochromatic Shadow Rays”.

The arrangement is such that the light flow illuminates a sloping piece of white cardboard. With this set-up successive stages in the transformation of the projected image, with increasing distance from the prism, are simultaneously displayed. Since the spatial distribution of the spectral phenomenon is essentially two-dimensional (in this case horisontally) we can use the vertical dimension to display successive stages, under the assumption that the different strata are all equivalent. Which presumes careful adjustment of the set-up.
Another pertinent remark. What we see and photograph is the luminance on points of the screen and
this reproduces the illumination; but it is the total illumination incident from various directions
to that point. So the image we see might be the superposition of several different images.
Anyway, the arrangement gives us an immediate view of the spatial distribution of the phenomenon. An aspect that particularly interested Goethe, who didn’t like to regard the spectrum as something once and for all “given” – or being built up from given elementary constituents.
§217 Bei allem diesem lassen wir niemals aus dem Sinne, das diese Erscheinung nie als eine fertige, vollendete, sondern immer als eine werdende, zunehmende und in manchem Sinn bestimmbare Erscheinung anzusehen sei.
If I introduce a thin stick, with a reflecting plane side, it creates through reflection an image: a thin bright line. We call it “a ray of light” because this is the way it looks. (But beware of imagining some invisible physical entity “behind” it. It is just a restricted beam of light from the collimator, illuminating the white cardboard along a straight line, visualizing “the principle of rectilinear propagation of light”, or better: the spatial directedness of illumination.) Simultaneously the stick casts a tiny shadow. A “dark ray” on a bright background.
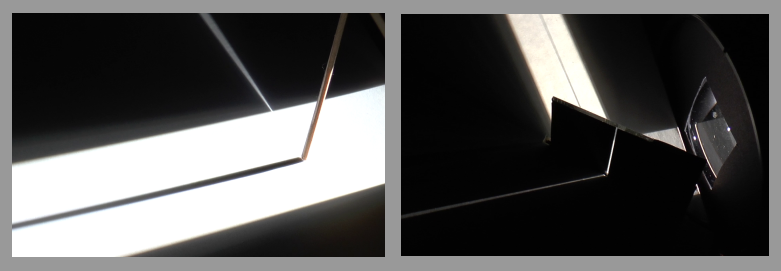
Likewise, we can put a slit-mirror in front of the collimator. The flow of light is split in two parts. One that passes through the slit, producing on the other side a bright line – a ”ray of light”. Simultaneously the reflected dispersed white light, from the surface of the mirror, contains a dark line, starting from the position of the slit. It lies near at hand to think that this “ray of darkness” is due to the absence of the light that is transmitted through the slit.
The two images – the bright line in darkness and the dark line in brightness are complementary. Superimposed they would give us back the original homogeneuos white illumination.
Primary and secondary spectra
Next step is to place the big prism in front of the collimator. We get a flow of white
dispersed light, illuminating the cardboard surface. The fact that it is dispersed shows
itself at the borders to darkness, where we now get the typical boundary spectra: colour
transitions between dark and bright areas. Either via deep blue and cyan or via deep red
and yellow. These are the two primary spectra (as Goethe saw it).

If I put the thin stick, with a reflecting plane side, in the way of the light it creates an image: a thin bright line, spreading out into a successively broader spectrum of colours. We call it a N-spectrum (after Newton). Simultaneously the stick casts a tiny shadow that spread out into an inverted spectrum. We call it a G-spectrum (with reference to Goethe, who was the first to stress its importance for the understanding of colour.)
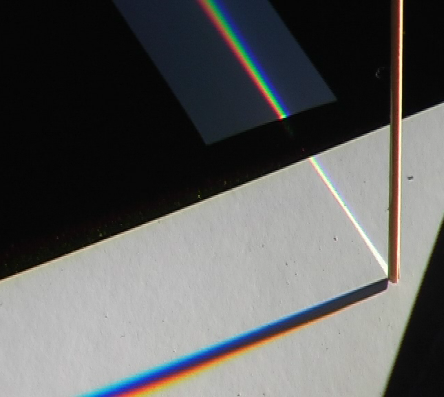
What in the earlier experiment with collimated light was a light-ray and a shadow-ray has now,
in dispersed light, become a light-spectrum and a shadow-spectrum. A bit more sophisticated
optical entities!
Likewise, as above, we can introduce a mirror, with a narrow slit on it. The illumination from
the colllimator is split in two parts. One that passes through the thin slit, producing on the
other side a line spreading out to a successively broader N-spectrum. And one that is reflected.
This one contains a dark line, starting at the slit on the mirror and successively widening into
an inverted spectrum, a G-spectrum. These are the two secondary spectra.
Complementarity
How can we experimentally “prove” that the N- and G-spectra are complementary, in the sense that
they add up to white? Answer: By superimposing them, which can be done with the help of a mirrors.
We use a slit-mirror and behind that an ordinary plane mirror. (See figure of the
arrangement. Black lines do not represent light-rays, only indicate the geometry
of the set-up. White is the reflecting side of the mirrors.)
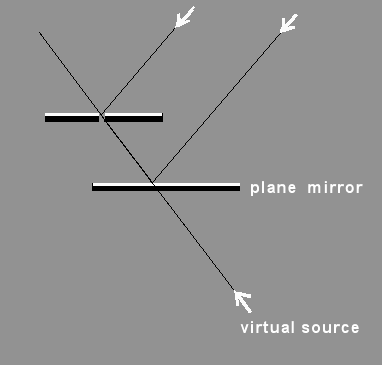
If the upper mirror is illuminated by the dispersed white light, but the lower mirror is not,
then a G-spectrum is produced in the light field uppwards.
If the lower mirror is illuminated by the dispersed white light, but the upper one is not,
then we get a N-spectrum upwards, in the darkness there.
If both mirrors are illuminated by the white dispersed light, then we get a homogeneous white
field above the upper mirror. Because the N- and the G-spectrum are superimposed. (It is the
same situation as if the upper mirror had been a mirror without a slit.)
Separating spectral parts
A spectrum is an optical image and as such a whole which cannot a priori be regarded as decomposable
into separately existing parts, without these “parts” changing their appearance. (Compare with a
coloured shadow image which may loose its hue if taken out of its context.) However, the spectrum
looks like an array of coloured lights put in a row side by side. A crafts-minded person may want
to try to separate these visual parts into physical, independent parts. Hence Newton´s idea of the
existence of different “sorts” of light constituting the spectrum, as well as being elementary
constituents of the flux of white light out of which the spectrum was produced.
Let the image of a N-spectrum fall on a screen with a narrow opening, so that for instance the
green part of the spectrum hits the opening. On the other side of the screen we get a green “ray”.
It seems that it can exist on its own, in dark space. But beware: it is still just an image. It is
even a question of consistency still to regard it as a spectrum. But it is a strongly modified
spectrum. It is a spectrum where all parts, except the green region, are very dark, with luminance
almost zero. But these missing parts of the spectrum can be as important as the present ones, for
instance in colour theory. We always have to consider the spectrum as a whole.
Theoretical excurse: The superposition principle
The reason why its is possible to divide the spectrum arbitrarily into parts is the property of
light that is expressed in the superposition principle of light intensities. Since
acient times this has been rgarded as one of the mysteries of light: that
variously directed illuminations do not collide or in any way interfere. Light
beams could just cross each other without disturbing each other. Images can be
located at the same place without taking up space, as material bodies would do.
I specifically
mention this principle, because it is of fundamental importance for the possibility of treating
the intensive aspect of light (the extensive aspect being the spatial, geometrical one). In other
words: it makes possible the science of photometry, with operational definitions of illuminance
and luminance as fundamentally measurable magnitudes.
When Isaak Newton speaks about the light of the Sun as a Mixture of Different Sorts of Light he
implicitly refers to, and makes use of, the superposition principle. But observe, according to
this principle you may equally well say that white light is a mixture of yellow and blue light.
Or a mixture of red, green and blue light (as on a computer screen). In the present investigation
it is a sufficiently good approximation to regard the dispersed white light as a superposition of
red, green and blue light with slightly different spatial directions. It simplifies the theoretical
understanding und does not prevent a later more precise treatment in terms of continuous spectra
and an infinite-dimensional vector space for the desccription of the intensive aspect of light.
Last steps towards the isolated purple ray
Next, we may ask: can we extract a monochromatic ray from the G-spectrum? For instance, select the
central purple part of it, separate it from yellow and cyan, and make it pass on as a solitary ray?
Again we set up a screen with a narrow opening on which the purple part of a G-spectrum is projected.
On the other side: we find no purple ray, but a red and a blue ray with a dark space in between.
This dark space could be regareded as a ray och darkness, a “dark-ray”. Ths dark-ray is just the
missing green of the N-spectrum. It is a kind of “zero-green ray”.

We can also think about it as follows. What we see on the other side of the screen is a N-spectrum,
and it is the N-spectrum characteristic of purple light. Each colour of the G-spectrum has its typical
N-spectrum.
What would it mean, really to lift out the purple component from a G-spectrum, analogously to how we
did it with the green part of the N-spectrum? The G-spectrum is created out of white light by a selective
darkening. It is embedded in white. But then even any variant of it must be surrounded by white. The
purple ray must appear as a shadow-ray in bright space. (Analogously with how the green ray from a
N-spectrum exists in dark space.)
How can we produce such an image: a purple ray surrounded by neutral white illumination?
The solution to this was found by the physicist Torger Holtsmark and described it as a thought experiment
in an article in American Journal of Physics, in 1970. The trick is again to use, instead of an aperture
screen, a plane mirror with a slit in. In fact, even two slit-mirrors, mounted parallell to each other.

Let the mirrors be illuminated by the dispersed white light. As before, a G-spectrum should be
generated in the reflected light. But that would be the case if the slit was dark. Now, instead,
it is purple, since we direct purple illumination towards the slit, from the back side of the mirror.
So we still see a G-spectrum, but a G-spectrum consisting of only the central part. The yellow and
cyan parts have been lifted up to white.
The fascinating thing with this purple ray is that it does not split up if passed through a second
prism, as long as we are in the dispersed white light-field. So from the appearance of the image
we had reason to call it a “monochromatic shadow-ray”.

II. The inverted spectra of red, green and blue-violet.
So far I have described what was already demonstrated in the video. Now follows the supplementary
observations.
What if we try to analyse rays from a Newtonian spectrum in terms of the inverted spectrum?
We can use almost the same setup, by a clever substitution of the central mirror. We use a
very narrow mirror, sending a Newtonian spectrum backwards.
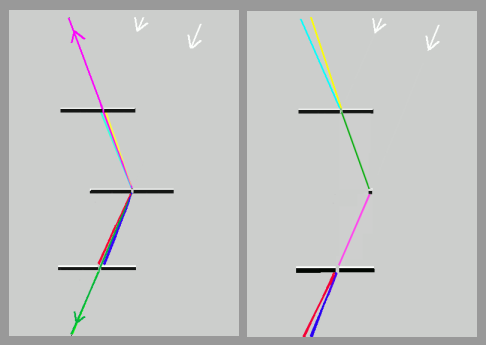
Thus we can test what happens if we send one of the elementary constituents of the Newton-spectrum
through the slit in the uppermost mirror (in the schematic figure), thereby letting it enter into
the bright field. Exactly as we did with purple, described above.
The following three pictures show the result. The green is spilt up into yellow and cyan. Red in
purple and yellow, blue-violet in cyan and purple. Which are the inverted spectra of these
lights.
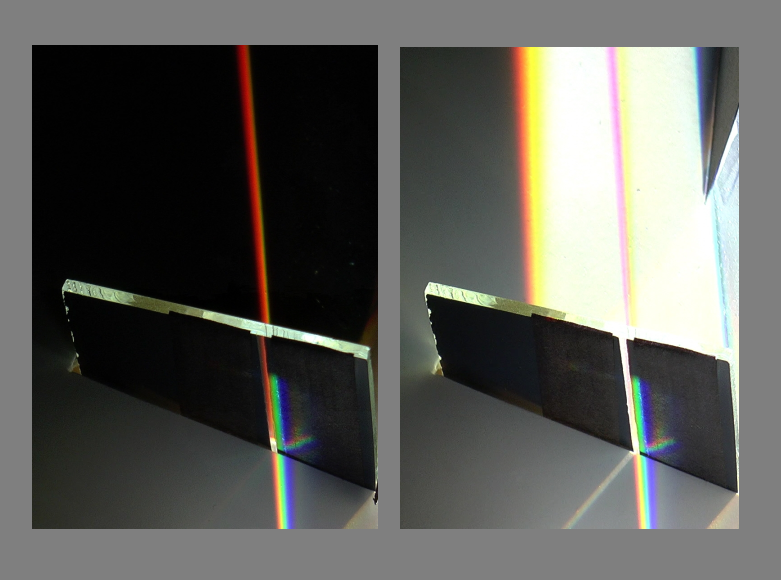
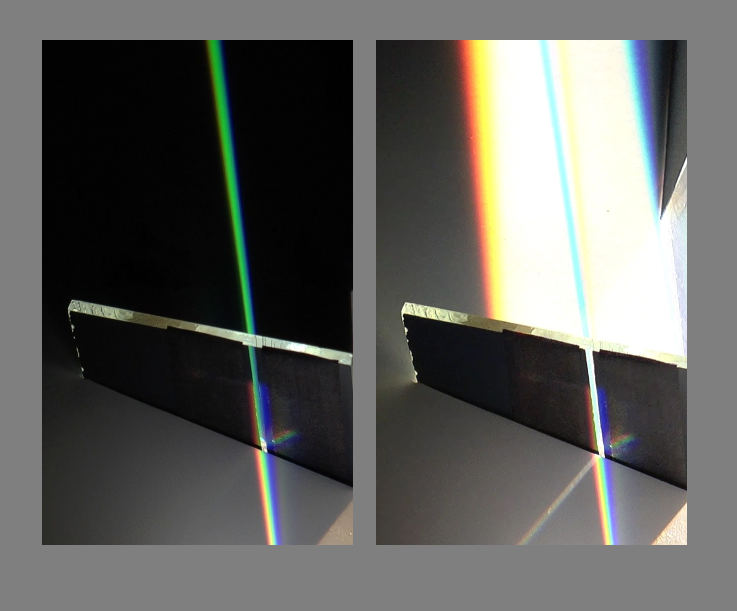

III. Analysis
So far I have shown how to produce Goethe-spectra of selected
parts of a Newtonian spectrum. Let us compare the two kinds of spectra for the
eight main colours:

Notice that the Goethe-spectra correspond closer to how ordinary illuminated colour samples look. The spectrum of a white
board is white. The spectrum of a magenta sample is magenta on white ground. Likewise
the spectrum of a yellow sample is yellow on white; of a cyan sample it is cyan
on white.
Notice the complementarity between the two kinds of spectra: For instance, the Goethe-spectrum of red and the Newton-spectrum of cyan added together should give white. And so would the n-spectrum of red plus the g-spectrum of cyan. We are dealing with two ways of representing the same colours.
The brilliance of both kinds of spectra, as shown above, is due to the fact that the slit opening on the mirror is quite large, relative to the total width of the spectrum. Almost 1/3. Look here:

This means that we are essentially studying coloured lights with ideal spectra. That is, the combinations of boundary spectra that Goethe studied, looking through a prism at a white square on black ground and a black square on white ground, respectively. Varying the distance, to make the boundary spectra overlap more or less.
The reason why the "rays" we produce look "monochromatic" -- in the sense that they have one single hue -- is partly to be found in the trichromacy of colour vision, which makes the Newtonian spectrum roughly look as if consisting of three parts: a red, a green and a blue.
It is interesting to note that even at this very low spectral resolution, the colour spectra still have so great colorimetric purity that they cannot be reproduced on an ordinary computer screen. They fall outside the colour gamut, spanned by the RGB-triangle. Which means that you have to follow Goethe's example and look through a prism, in order to se how the spectra look in reality.
If by using narrower slits we should try to produce even purer spectra, representing "homogeneous light" (in Newton's sense), then the colours of the Goethean spectra would lose contrast and soon turn into white. Simultaneously the colours of the Newtonian spectra would lose brightness, but keep their purity and still be observable in a dark environment.
Newton made his observations in a dark chamber and concentrated his efforts on trying to isolate what he considered might be the ultimate constituents of sunlight. He had a different intention with studying prismatic spectra than Goethe had. One might say: he was trying to find a procedure to make certain properties of light measurable.
Goethe's interest was colour perception. Hence he was fascinated by the lawful variations of possible spectra, obtainable under systematically varied conditions. Preferably studied in daylight.
A technical note
The relative brightness of the light reflected on the mirror and the light coming from behind through the slit is crucial, to get the pure results shown in the figures. This is because the complementary condition must be fullfilled. (That the sum of the luminances of the two superimposed spectra should be constant and equal to the luminance of the surrounding area.) In our particular case the light onto the mirror needed a 50% reduction, with help of a Kodak Wratten neutral filter ND = 0.30 in order that the inverted spectrum should not be too dominant.
The photographic documentation was made by help of a Panasonic HC-X920, in manual mode with careful selection of the most adequate colour balance and aperture.
Järna, July 20 and September 27, 2016 Pehr Sällström
last rev 2020-06-01